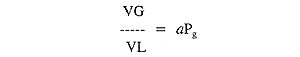SCUBA DIVING EXPLAINED Questions and Answers on
Lawrence Martin, M.D. Copyright 1997
|
|||||||||||||||||||||||
|
Home Brief History of Diving Glossary
|
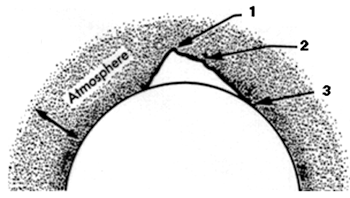
SECTION DAn Explanation of Pressure and the Laws of Boyle, Charles, Dalton, and HenryWHAT IS PRESSURE? Key to understanding scuba diving is the concept of pressure, and how it varies with depth. We intuitively understand that pressure is some type of force, but how is it actually defined? Pressure is a force or weight per unit area. All matter, including air, has weight due to earth's gravity. Accordingly, anything exposed to air is under pressure - the weight of the atmosphere above it. This weight of air, due to gravity, is known as atmospheric pressure (Figure 1).
Figure 1. Earth is surrounded by a layer of atmosphere which is densest at sea level; the atmosphere becomes thinner - less dense - with altitude. Figure is not drawn to scale. From experience just carrying a container of water, we also know that water is much heavier than air. Since pressure is related to weight, water pressure must be far greater than air pressure, and of course it is. Gravity keeps the atmosphere wedded to the earth. Without gravity, earth's atmosphere would float away to outer space. Since gravity diminishes with distance from the center of the earth, air weighs less at altitude than at sea level. Literally, air at altitude is "thinner" (meaning less dense) compared to sea level, and air becomes progressively less dense with increasing altitude (Figure 1). A cubic foot of air on the summit of Mt. Everest contains only about a third as many molecules as a cubic foot at sea level, and hence weighs only about a third as much (Figure 2). (Everything weighs less at altitude, including people, but we don't notice the difference except in outer space. You would not feel lighter on the top of a high mountain. In outer space, miles from earth's center of gravity, weightlessness is experienced.)
Figure 2. A cubic foot of air at the summit of Mt. Everest contains only about a third the number of molecules as a cubic foot at sea level, and hence weighs a third as much. WHAT IS AIR PRESSURE? Air pressure can be specified in several ways; the most popular term used in scuba diving is "pounds per square inch" or "psi." At sea level the pressure exerted by the atmosphere is 14.7 psi. "Per square inch" refers to the surface area subjected to the weight of the air above it; the units could just as well be per "square foot," "square yard" or "square meter," but then the numbers would be correspondingly higher. Stated another way, a column of air one inch square and about 50 miles high (a distance that encompasses virtually all of earth's atmosphere) weighs just 14.7 pounds (Figure 3). Not a lot of pounds for something fifty miles high, but not exactly weightless either. Most of the atmosphere's weight is actually contained in the first few miles, where gravity exerts its greatest effect. An air column one-inch square, from sea level and extending 3.4 miles high (18,000 feet), weighs about half that of the full air column, or 7.35 pounds. The remainder of the one-inch-square air column, from an altitude of 3.4 miles to outer space, weighs another 7.35 pounds.
Figure 3. A one-square-inch column of the entire atmosphere weighs 14.7 pounds; this is the atmospheric pressure at sea level. Since half the weight occurs in the first 18,000 feet (3.4 miles), air pressure at this altitude is one half the total, or 7.35 psi.
TEST YOUR UNDERSTANDING Answers To further consider the concept of air pressure, open your hand palm upward. The palm surface of an average-sized hand (with fingers closed) covers about 25 square inches. Now lift your hand quickly upward with the palm flat out (Figure 4). Assuming you are at sea level, and your hand is average-sized, you are lifting 25 x 14.7 or 368 pounds of air! Why, then, does your lifting seem so effortless? If you had to lift 368 pounds of anything with one hand you couldn't do it. It feels effortless because air pressure is evenly distributed around your hand, and the molecules of air are easily movable. At sea level, air pressure is 14.7 pounds per square inch on top of your hand, underneath your hand, and on all sides. Thus you don't really 'lift' 368 pounds, though that is the weight of air on top of your hand. As you lift your hand you move some air molecules out of the way and other molecules immediately come under and around your hand. The pressure surrounding your hand stays the same: 14.7 psi. Because the pressure is evenly distributed, you don't feel any weight in lifting your hand. (There is resistance, however, as it takes time for air molecules to get out of the way. Resistance in the face of equal pressures is much better appreciated under water, since water is much denser than air.)
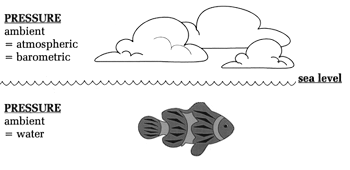
Figure 4. Air pressure around your hand - or any object in the atmosphere - is evenly distributed. WHAT IS THE DIFFERENCE BETWEEN AMBIENT, BAROMETRIC AND ATMOSPHERIC PRESSURE? It can be confusing when different terminology is used to describe the same thing. One example is the use of multiple terms to indicate the pressure around us and the different measurement units for that pressure. The surrounding pressure, on land or under water, is referred to as the ambient pressure. If the surrounding pressure is from the weight of air, it is the atmospheric pressure. If the surrounding pressure is from the weight of water, it is the water pressure (Figure 5).
Figure 5. Ambient Pressure on land and under water. When surrounded by air, ambient pressure = atmospheric pressure = barometric pressure. When surrounded by water, ambient pressure = water pressure. Weather forecasters usually report air pressure in terms of a barometer reading in inches, e.g., "the barometer is currently 30 inches of mercury and rising." A barometer is an instrument for measuring atmospheric pressure, so barometric pressure is just another term for atmospheric pressure. WHAT ARE THE MEASUREMENT UNITS FOR THE AMBIENT PRESSURE? There are several units of measurement for ambient pressure. None is universally used, as different groups seem to prefer different terms to describe this pressure. The various terms are listed in Table 1, arbitrarily subdivided according to whether or not they are commonly employed in diving. Note that "bar" is commonly used in European diving. North Americans who rent air gauges in other countries may find them calibrated in bars. Thus a tank filled to 3000 psi would register 206 bars.
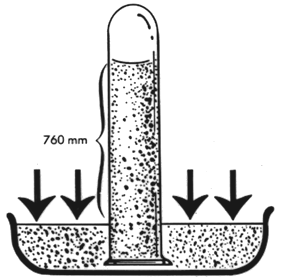
WHAT IS AN "ATMOSPHERE" OF PRESSURE? One atmosphere (atm.) is the air pressure at sea level and equals 14.7 psi. Note that the term "one atmosphere" is just a measurement; you don't have to be at sea level to be surrounded by one atmosphere. You could be in a submarine 330 feet under water and still be surrounded by one atm. of pressure (though the submarine hull would be surrounded by 10 atm. of water pressure). Similarly, two atm. is twice the sea level pressure. Two atm. = 29.4 psi, a pressure reached at 33 fsw (Table 1). You could also experience this pressure on land, in a hyperbaric chamber. Conversely, the air pressure at 18,000 feet altitude = 7.35 psi, but this could be experienced at sea level as well, inside a chamber that can simulate altitude. Don't confuse "atmosphere," which is a unit of measurement, with "atmospheric pressure," which is a general term for the surrounding air pressure. Atmospheric pressure could be any value, e.g., one atm. (sea level pressure), one-half atm. (18,000 feet), zero (outer space), or three atm. (inside a hyperbaric chamber). Another important unit of measurement is millimeters of mercury, abbreviated mm Hg (Hg is the chemical symbol for mercury). Some texts refer to mm Hg by the term "torr," after the Italian Evangelista Torricelli (1608-1647), a pioneer in the measurement of atmospheric pressure; one mm Hg = one torr. Air pressure at sea level is 760 mm Hg (or 760 torr). In medicine and science, mm Hg is commonly used as the unit for partial pressures of gases. Note that psi and mm Hg reflect different ways of measuring the same thing. At sea level air weighs 14.7 pounds per square inch of earth's surface, so the pressure is 14.7 psi. It is also true that this weight of air will support a column of mercury 760 mm high (Figure 6), so the air pressure is also 760 mm Hg. I also mentioned the weather forecaster's lingo, e.g., "the barometer is 30 inches of mercury and rising." Inches and millimeters are both measurements of length. In the United States, non-scientific measurements remain largely non-metric (many people would like to change that!). In most of the rest of the world the metric system is used for all measurements. Metric lengths are described in millimeters, centimeters, meters and kilometers. Table 2 lists some common units of measurement for average atmospheric pressure, shown for various altitudes. WHAT IS THE DIFFERENCE BETWEEN ABSOLUTE PRESSURE AND GAUGE PRESSURE? Sea water weighs about 64 pounds per cu. foot (the exact weight depends on the quantity of dissolved salt, which varies slightly in the world's oceans). Using this value, 33 cu. feet of water weighs 33 x 64 = 2112 pounds. Dive to 33 feet depth, lay horizontal, and you will have 2112 pounds of water over every square foot of your body; this comes to 14.7 pounds per square inch, which is the atmospheric pressure at sea level. At 33 feet depth a diver is under two atmospheres of pressure: one atmosphere from the air above sea level and a second atmosphere from the 33 feet of water. The fact that 33 feet of sea water equals the pressure of the entire atmosphere is important in understanding the term "gauge pressure." Gauge pressure, discussed in some diving books, is the water pressure read by gauges that arbitrarily set sea level pressure to zero. Such instruments read the pressure at 33 feet depth as "1 atm. gauge," meaning that the pressure from water alone is 1 atm. However, if the dive commenced at sea level, then at 33 feet the actual, real, absolute pressure on the diver is two atmospheres, one from earth's atmosphere (14.7 psi) and another from the water (also 14.7 psi). Unless you have one of these gauges don't be concerned about gauge pressure. You need to know the actual or absolute pressure as you dive. If you commence your dive at sea level the absolute pressure at 33 fsw is two atmospheres; at 66 fsw, three atmospheres; at 99 fsw, four atmospheres; etc.
Figure 6. The weight of air at sea level will support a column of mercury 760 mm high. In a simple experiment shown by this figure, a tube longer than 760 mm, and closed at one end, is filled with liquid mercury. The tube is then inverted so that its open end is submerged in a pan of mercury, at which point mercury in the tube falls to a certain height. The height of the column of mercury in the inverted tube equals the pressure of air over the open pan of mercury. At sea level the column of mercury is 760 mm high, so 760 mm Hg is the pressure of air at sea level.
TEST YOUR UNDERSTANDING Answers WHAT CAUSES CHANGES IN ATMOSPHERIC PRESSURE? There are 25.4 millimeters to the inch, so a barometric pressure "30 inches of mercury" is the same as "762 millimeters of mercury." That amount of atmospheric pressure will support a column of mercury 30 inches or 762 mm high. The terms "rising" and "falling" as applied to barometric pressure refer to minor changes in atmospheric pressure with changes in weather. A "high pressure front" is one where the barometric pressure for a mass of air is slightly higher than average. Although "high and low pressure" fronts may have profound consequences for the weather (e.g., a low pressure front portends bad weather), the pressure changes, per se, have little effect on people; the variations are usually too small to be of any significance. Barometric pressure at a specific location (e.g., the airport at Denver, the beach at Waikiki, the top of the Empire State Building) seldom fluctuates by more than 30 mm Hg (1.2 inches Hg) throughout the year. There is, of course, an average barometric pressure for any given location. At sea level throughout the world this average barometric pressure is 760 mm Hg. Fluctuations in barometric pressure at a specific location, used to predict the weather, are of course different from changes in barometric pressure that come about from changes in altitude or depth. Changes in pressure with altitude have nothing to do with the weather, but are due to the change in weight of air above you. The closer to the earth's surface, the greater the weight of air above you and the greater the air pressure. Similarly, pressure changes in water are solely related to the change in weight of water above you; the deeper you dive, the greater the weight of water above you and the greater the water pressure.
TEST YOUR UNDERSTANDING Answers WHAT IS THE COMPOSITION OF AIR? Air is a mixture of gases, mainly oxygen (21% by volume) and nitrogen (78% by volume). The other 1% of air is made up of several other gases such as carbon dioxide (CO2), argon, krypton and neon. The actual percentage composition of dry air (what scuba divers inhale) is shown in Table 3. For scuba diving purposes it is convenient to consider nitrogen as 79% of the air, since the other inert gases (principally argon) must also be considered in computing decompression schedules. Thus you will find many texts listing nitrogen as "79%" of the air. In any mixture of gases (e.g., air), the individual gases don't chemically combine with each other. The gases maintain their individual identity and percentage regardless of how much or little pressure the mixture is subjected to. The percentages of gases shown in Table 3 are the same throughout the breathable atmosphere. They are also the same inside a tank of compressed air and in the air as it emerges from the tank on its way to the diver's lungs, regardless of depth. This fact takes on critical importance as water pressure increases with increasing depth because, although the percentages are unchanged, the total pressure exerted by each gas component increases proportionately. The increases in component gas pressures account for some of the major problems inherent in compressed air diving: nitrogen narcosis, decompression sickness and oxygen toxicity (see Sections G and I).
WHAT ARE THE GAS LAWS AND WHY ARE THEY IMPORTANT TO DIVING? So far we have discussed pressure as it relates to air in the atmosphere. Scuba divers, of course, are interested in what happens to air under water. Air under water obeys the same laws as air in the atmosphere. I will introduce the gas laws in this section and, in Chapter 5, use them to further explain physiology under water. The four important gas laws are those of the Englishmen Robert Boyle (1627-1691), John Dalton (1766-1844), and William Henry (1774-1790), and the Frenchman Jacques Charles (1746-1823). The idea is not to belabor laws of physics, but to emphasize relationships that explain what happens when you dive. The four gas laws are useful because they predict changes in air pressure, volume and temperature as compressed air divers descend and ascend. WHAT IS BOYLE'S LAW? Boyle's law states: At constant temperature, the volume of a gas varies inversely with the pressure, while the density of a gas varies directly with pressure. Simplified: If temperature is kept constant, as air pressure increases the volume of a gas decreases, and vice versa. Mathematically,
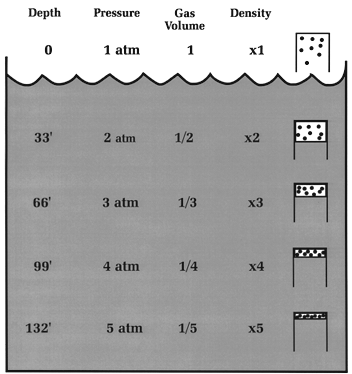
where P and V are the pressure and volume, respectively, and K is a constant. Change P, and V will change in the opposite direction, so that their product is maintained at a constant value. Now let's illustrate this law. Suppose you have a container open on one end that is inverted over water; as the container is lowered in the water the trapped air will be compressed by the water pressure (Figure 7). Assume the container holds one liter of air at sea level pressure (one atmosphere). PV = 1 liter x 1 atm. = 1. Increase the air pressure to 2 atmospheres and Boyle's law predicts the volume of air in the container will be 1/2 liter (Figure 7).
Figure 7. A container open on one end has one liter of air at one atmosphere. The air is compressed by taking it under water. Boyle's law predicts that at two atmospheres pressure (33 fsw) the volume of air in the container will decrease by one half and the density of air will double. At 3 atmospheres pressure, the volume of air will be 1/3 of that at sea level; and the density triples; etc. Note that Boyle's law also relates to gas density. Increase the pressure of a fixed volume of gas and the density increases, and vice versa. This part of the law becomes particularly important on deep dives; it predicts that the inhaled air will become denser the deeper one goes. As a result of increasing air density, deep divers often notice greater difficulty breathing. Without doubt Boyle's is the most important law in scuba diving (but not the most important rule, which is never hold your breath). All certification courses stress Boyle's law. Don't leave home without it!
TEST YOUR UNDERSTANDING Answers WHAT IS CHARLES'S LAW? Charles's law states: At a constant volume, the pressure of gas varies directly with absolute temperature. Simplified: Given a constant volume of gas, the higher the temperature the higher the gas pressure, and vice versa. Mathematically, Compared to Boyle's law, Charles's law is not as important for scuba divers because temperature under water seldom changes enough to seriously affect air pressure. However, the law is useful to keep in mind when filling air tanks, especially when there is a large difference between air and water temperatures. Suppose you have a steel scuba tank holding 80 cu. ft. of air at a pressure of 3000 psi, filled when the air temperature was 90�F. Now you take the tank into water that is 75�F. Before you take your first breath of that tank's air, Charles's law predicts that the tank pressure will be...what? Lower than 3000 psi. Since T2 is less than T1, the law predicts that P2 will be less than P1. (To know how much less you need to convert T1 and T2 into absolute temperatures by adding 460 to each fahrenheit temperature.) Scuba shop proprietors know about Charles's law, which is why they often fill tanks in a water bath where the temperature is about the same as the ocean (or wherever the diving will take place).
TEST YOUR UNDERSTANDING Answers WHAT IS DALTON'S LAW? Dalton's law states: The total pressure exerted by a mixture of gases is equal to the sum of the pressures that would be exerted by each of the gases if it alone were present and occupied the total volume. Simplified: The pressure of any gas mixture (e.g., air) is equal to the sum of pressures exerted by the individual gases (e.g., oxygen, nitrogen, and each of the minor gases). Mathematically, where PTOTAL is the total pressure of a gas mixture (e.g., air), and P1 and P2 are the partial pressures of component gases (e.g., oxygen and nitrogen). The term POTHER is used to signify partial pressures of all other gases in the mixture. WHAT IS PARTIAL PRESSURE? Partial pressure is a very important concept in scuba diving, in part because it aids in understanding Dalton's law. Partial pressure is the pressure exerted by an individual gas, whether that gas is part of a mixture (such as air) or dissolved in a liquid (such as blood) or in any body tissue. Partial pressure of a gas (PG) is determined by the fraction of the gas in the mixture (FG) times the total pressure of all the gases (excluding any water vapor present):
In air at sea level, the partial pressures of oxygen and nitrogen are:
Note that we could have used any of the terms in Table 1 (page 58) to denote partial pressure. Thus, in mm Hg the partial pressures of oxygen and nitrogen at sea level are (assuming no water vapor):
TEST YOUR UNDERSTANDING Answers WHAT DOES DALTON'S LAW PREDICT ABOUT CHANGES IN PARTIAL PRESSURE WITH CHANGES IN AMBIENT PRESSURE? (If you answered questions 13 and 14 correctly you already know the answer to this question.) The percentage of gases making up air is the same throughout the breathable atmosphere. Regardless of altitude, the composition of air is about 21% oxygen, 78% nitrogen, 1% other. Similarly, even though air is compressed in a scuba tank, the percentage of the individual gas components is the same. Given this fact and Dalton's law, we see that as air pressure increases or decreases, the partial pressure of each gas will do the same. With increasing altitude, for example, the partial pressure exerted by each gas in the air will decrease. With increasing depth, the partial pressure exerted by each gas in the air we breathe will increase. The lower partial pressures at altitude reflect the fact that there are less molecules of O2 and N2 per volume than at sea level. At the highest point on earth, the summit of Mt. Everest, air still contains 21% oxygen and 78% nitrogen but the number of oxygen and nitrogen molecules per volume of air is only about 1/3 that at sea level (Figure 2); thus the partial pressures of oxygen and nitrogen are only 1/3 those of sea level. People who fly in unpressurized airplanes, or engage in mountain climbing or hot air ballooning, subject themselves to a decrease in air pressure as they ascend, and an increase in air pressure as they descend; the opposite, of course, happens to scuba divers. Since air at altitude contains fewer O2 molecules, a passenger plane that flies above a certain altitude must pressurize its cabin to the air pressure of a lower altitude than actually flown. When you fly at 30,000 feet, for example, the cabin is pressurized to an altitude of about 7000-8000 feet. At 7000 feet altitude, but not 30,000 feet, the air contains enough oxygen molecules for comfortable breathing at rest (albeit fewer than at sea level). If the plane suddenly lost its artificial cabin pressure at 30,000 feet and did not descend immediately to a safer altitude, and there was no way to get extra oxygen to the passengers and crew, everyone aboard the plane would soon lose consciousness. If the problem remained uncorrected everyone would eventually succumb to hypoxia. As a practical matter people can live and work up to about 16000 feet; beyond that altitude hypoxia makes it difficult to breathe for long periods, particularly with any exertion. However, people have actually climbed to the summit of Mt. Everest without breathing extra oxygen, a feat of incredible endurance (some would say incredible foolishness). Two principle adaptations allow people to function at high altitude: increased blood volume (hemoglobin carries most of the blood oxygen, so the more blood the more of the scarce oxygen molecules can be taken up from the thin atmosphere); and hyperventilation, which is the medical term for over breathing. Hyperventilation lowers the blood carbon dioxide level and allows the mountain dweller to bring more oxygen molecules into the lungs. Hyperventilation is much more effective in adding oxygen to the blood when the blood is low in oxygen than when it is normally supplied. (While hyperventilation is a positive adaptation for altitude, it can cause problems in divers, both breath-hold and scuba; see Chapter K.)
TEST YOUR UNDERSTANDING Answers WHAT IS HENRY'S LAW? Henry's law states: The amount of any gas that will dissolve in a liquid at a given temperature is a function of the partial pressure of the gas in contact with the liquid and the solubility coefficient of the gas in that particular liquid. Simplified: As the pressure of any gas increases, more of that gas will dissolve into any solution with which it is in free contact. Mathematically, Taken together, Henry's and Dalton's laws predict two very important consequences: 1)When ambient pressure is lowered (as at altitude), the partial pressure of oxygen and nitrogen in the body must fall, and there will be less molecules of each gas dissolved in the blood and tissues. 2)When ambient pressure is raised (as when diving), the partial pressure of oxygen and nitrogen in the body must rise, and there will be more molecules of each gas dissolved in the blood and tissues. The second statement is the physiologic basis for three important problems associated with compressed air diving: decompression sickness, nitrogen narcosis, and oxygen toxicity. These conditions are discussed in Sections G and I.
Answers to TEST YOUR UNDERSTANDING 1. c. Air pressure at sea level is 14.7 psi, which means the total column of air from sea level to outer space one inch square weighs 14.7 lbs. Since a square foot contains 12 x 12 = 144 square inches: 144 sq. in. x 14.7 lbs./sq. inch = 2116.8 lbs. 2. c. A square yard contains 9 sq. feet, or 9 x 144 sq. inches. Hence the column of air over a sq. yard weighs 9 x 144 x 14.7 = 19,051.2 lbs. 3. True 4. True 5. True 6. False 7. At 132 fsw absolute or total ambient pressure = 5 atm. Gauge pressure (pressure of water alone) = 4 atm. 8. b. 1.75 atm. 9. 253 mm Hg - summit of Mt. Everest 640 mm Hg - Denver 747 mm Hg - Miami 1520 mm Hg - inside hyperbaric chamber 10. Volume at 165 feet = 1 liter; density 6 times as great 11. a. The tank is a rigid structure. The compressed air is unaffected by ambient pressure until it leaves the tank. 12. b. 13. True 14. True 15. At 66 fsw, PO2 = .63 atm.; PN2 = 2.37 16. Partial pressure of oxygen is .21(380), or about 80 mm Hg. (This answer assumes dry air; any water vapor in the air will slightly lower the partial pressures of the other gases.) 17. PO2------0.84-----------0.21------------.105 18. c, f, and j are false; the other statements are true. REFERENCES AND BIBLIOGRAPHY Quoted sources and general references are listed by section or sections, in alphabetical order. An asterisk indicates references that are especially recommended. Medical textbooks and journal articles can be obtained from most public libraries via inter-library loan. For a list of companies that distribute free catalogs of diving books and videos, see Section U. SECTION D. An Explanation of Pressure and the Laws of Boyle, Charles, Dalton, and Henry For the sport diver, the training manuals of NAUI, PADI and SSI provide much useful information on physics and physiology of diving, as well as on all the diving skills. In addition, the following six books are highly recommended reference works for those who wish to read further on dive physics and physiology; the last three are textbooks marketed mainly to physicians and other medical professionals. *Hornsby A, Brylske A, Shreeves K, Averill H, Seaborn C. Encyclopedia of Recreational Diving. International PADI, Inc., Santa Ana, CA.; 1989. *NOAA Diving Manual, 3rd Edition. U.S. Department of Commerce, National Oceanic and Atmospheric Administration, Washington, D.C.; 1991. *U.S. Navy Diving Manual, Vol. 1 (Air Diving) and Vol 2 (Mixed-Gas Diving), Best Publishing Co., Box 30100, Flagstaff, AZ; 1993. *Bennett P, Elliott D, editors. The Physiology and Medicine of Diving. 4th edition. W.B. Saunders Co., Philadelphia; 1993. *Bove AA, Davis JC, editors. Diving Medicine. 2nd Ed., W.B .Saunders., Philadelphia; 1990. *Edmonds C, Lowry L, Pennefather J. Diving and Subaquatic Medicine. Butterworth Heinemann, Oxford; 1992. See references for Section E. |